Quadratic and cubic graphs are essential concepts in mathematics, especially in algebra and calculus. Understanding their properties can help solve various types of equations and real-world problems more easily.

RESOURCES

GCSE Maths – Types of Graphs Information Poster – Teach & Learn – Display, Decoration, Bulletin Board Poster – Paper Laminated – A2 42 x 59.4 cm (https://amzn.to/3PoceBC)

This is an affiliate link. If you click the link and buy the product, then the blogger gets a percentage of the sale or some other type of compensation. Prices are not different if you use these affiliate links. You will not pay more by clicking through to the link.

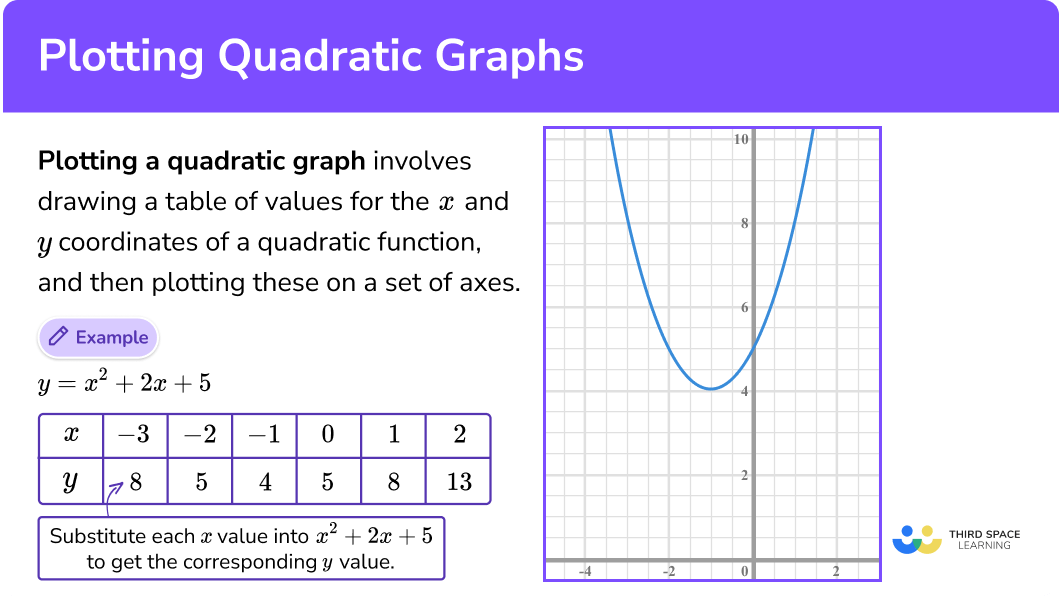
QUADRATIC GRAPHS

Quadratic functions are characterized by the term x², making it the highest power of x in the equation. These graphs form a shape known as a parabola. When the coefficient of x² is positive, the parabola opens upwards, creating a U-shape. Conversely, if the coefficient of x² is negative, the parabola opens downwards, leading to an n-shape. This basic understanding allows us to predict the behavior of quadratic equations and their graphs.

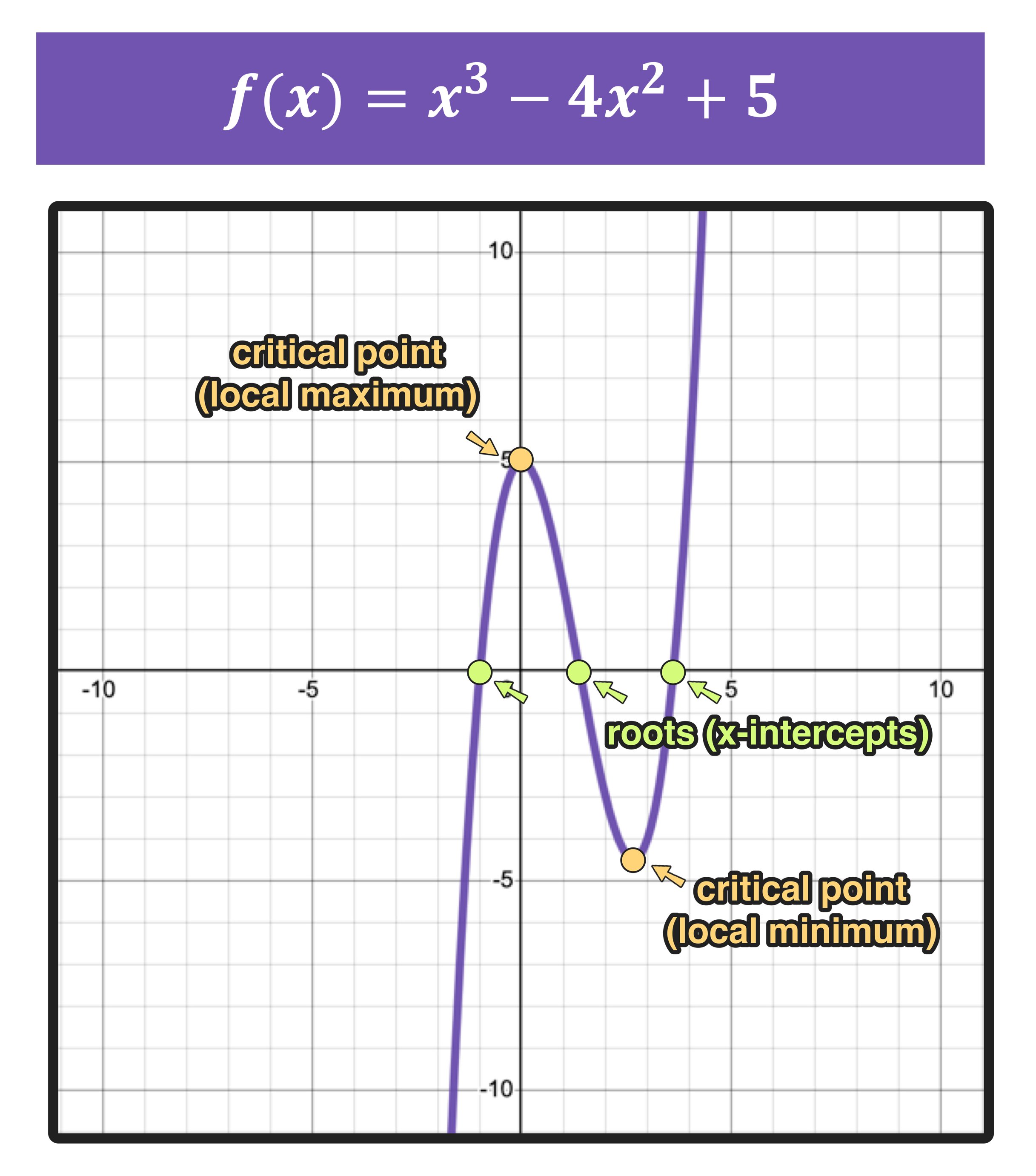
CUBIC GRAPHS

Cubic functions are defined by the term x³, which represents the highest power of x in the equation. The graphs of cubic functions all have a characteristic curve that features a distinctive wiggle. With a positive coefficient of x³, the graph starts from the bottom left and rises to the top right. In contrast, a negative coefficient of x³ results in a graph that starts from the top left and drops to the bottom right. This pattern helps us understand and visualize how cubic equations behave.

CONCLUSION

Comparing quadratic and cubic graphs highlights notable differences in their shapes and behaviors. While quadratic graphs always form a similar U-shape or n-shape, cubic graphs exhibit more complexity with their characteristic wiggle. Recognizing these differences can make tackling algebraic problems that involve these functions much more manageable.