In mathematics, the concept of inequalities is fundamental. It tells us about the relative size of two values. Think of it as the math version of the ‘less than’ and ‘more than’ we use in everyday life. Inequalities aren’t just about numbers; they’re about relationships. They help us understand conditions for ranges of values, not just fixed points.
The symbols for inequalities are straightforward: ‘>’, ‘<‘, ‘ What do these symbols mean? Simply put, ‘>’ means one value is greater than another, ‘ How do you tackle an inequality? It’s much like solving an equation. The golden rule remains the same: whatever operation you do to one side, you do to the other. But remember, reversing the inequality sign is crucial when you multiply or divide by a negative number.
Solving inequalities might seem daunting, but it need not be. I’ll walk you through the process so you can approach these problems with confidence. Let’s say you have an inequality, x + 3 > 5. To find x, you subtract 3 from both sides, giving you x > 2. It’s that simple.
RESOURCES
These are affiliate links. If you click a link and buy the product, then the blogger gets a percentage of the sale or some other type of compensation. Prices are not different if you use these affiliate links. You will not pay more by clicking through to a link.
QUADRATIC INEQUALITIES
While simple inequalities can be quite straightforward, quadratic inequalities require a bit more finesse. These contain an x2 term and can have two solutions that mark a range of possible values. Solving these typically involves graphing the related quadratic equation and identifying the values of x that satisfy the inequality.
Recognizing the crossover between equations and inequalities is pivotal. Where they differ is the solution: equations have a definite answer, while inequalities provide a range of answers. This distinction is critical in fields like engineering, economics, and sciences where precision matters and the stakes are often high.

GRAPHING INEQUALITIES
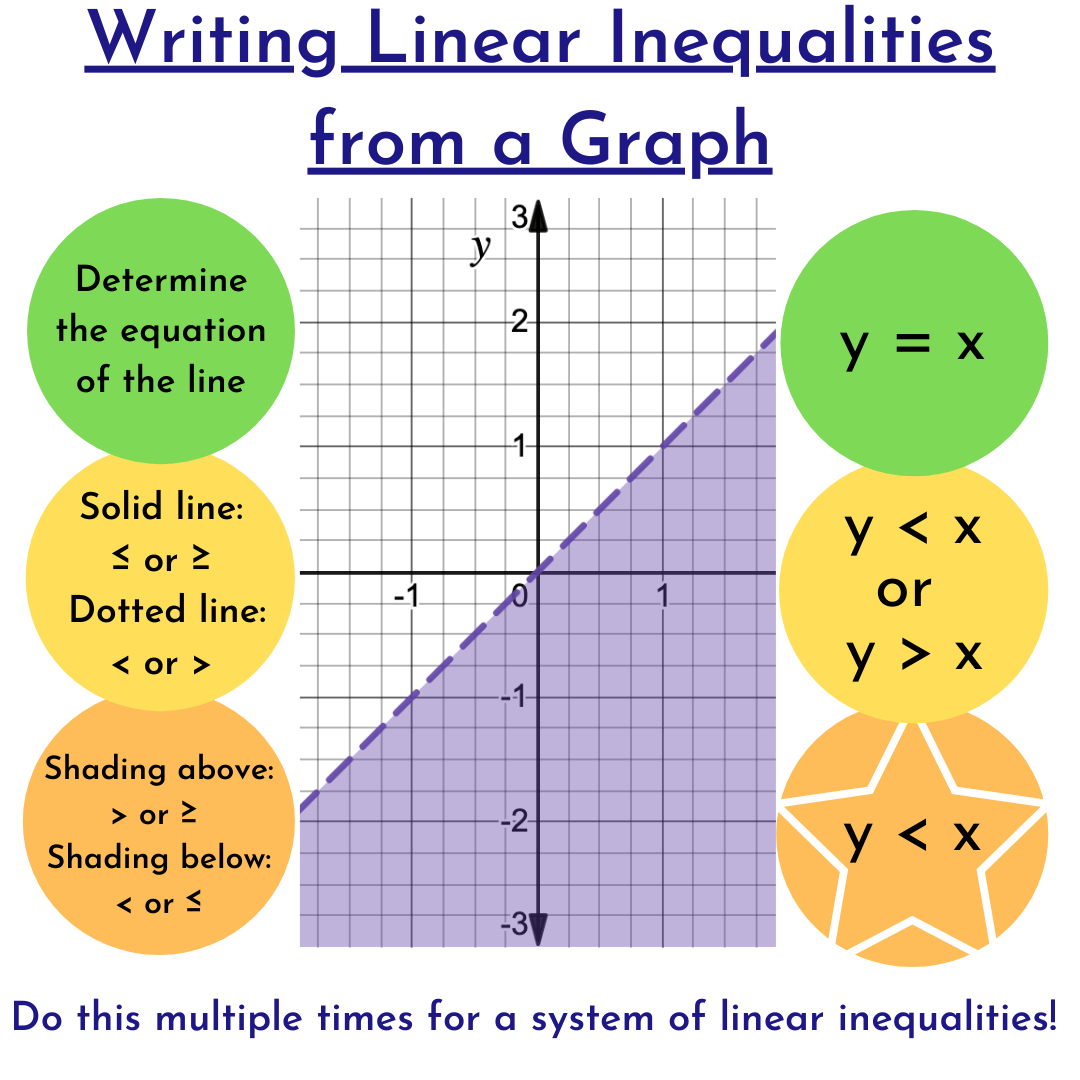
Graphing inequalities is more than a theoretical exercise. It’s a visual method that helps you understand the regions where inequalities hold true. When you see a graph with a shaded area, that’s the ‘where’ — where all the solutions to your inequality live. Converting an inequality to an equation and plotting it provides the ‘what’ — the boundary line. Shading tells you ‘how much’ or ‘how little’ of something you’re looking for.
LINEAR PROGRAMMING
But where do these graphs take us in the real world? This is where linear programming enters. Imagine you’re a business owner trying to maximize profit or a dietician planning a meal. Linear programming is your go-to tool. It uses inequalities to define constraints and optimizes a quantity — be it profit, nutrients, or something else entirely.
The technique is simple yet powerful. You sketch the inequalities as regions on a graph. The intersections of these regions form a shape, often a polygon. The ‘best’ solutions — the ones you’re really after — are found at the vertices of this shape. That’s the essence of linear programming: turning the complex into something decidedly simple and actionable.
Yes, this method is adored by economists and analysts for a reason: it provides CLEAR answers to complex optimization problems. Linear programming is a beacon in the decision-making darkness, guiding you to the point of maximum efficiency or minimum cost.
CONCLUSION
To sum up, inequalities aren’t just numbers and symbols on paper. They are a fundamental part of the toolkit used to make informed decisions in various sectors. By comprehending and applying the principles of graphing, quadratic inequalities, and linear programming, you can find solutions that are not just mathematically correct but also practically optimal.


No responses yet