RESOURCES
This is an affiliate link. If you click a link and buy the product, then the blogger gets a percentage of the sale or some other type of compensation. Prices are not different if you use these affiliate links. You will not pay more by clicking through to a link.
TERM TO TERM RULES
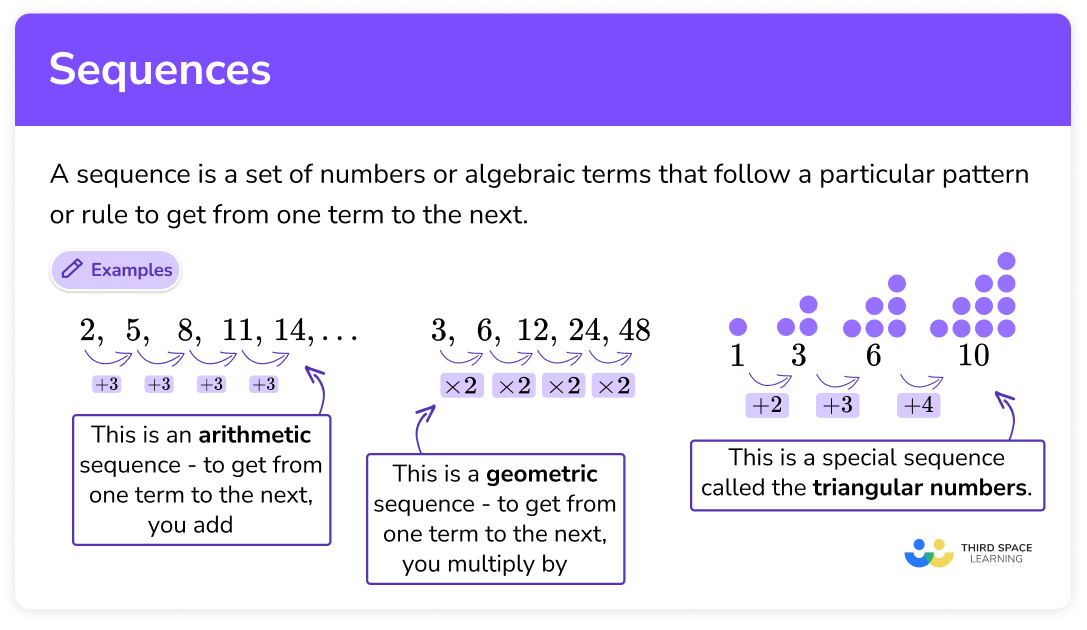
Sequences are everywhere. They are lists of numbers or shapes that follow a particular rule. Each item in the list is called a term. Knowing how sequences work can help with everything from solving math problems to understanding patterns in the world around us.
One of the most common types of sequences is the arithmetic sequence, or linear sequence. In these sequences, the difference between consecutive terms is always the same. For instance, in the sequence 3, 6, 9, 12, each term increases by 3. This consistent change makes arithmetic sequences pretty straightforward.
Another type is the geometric sequence, also known as a geometric progression. Here, consecutive terms are found by multiplying or dividing by the same value, called the common ratio. For example, in the sequence 2, 4, 8, 16, each term is multiplied by 2. Geometric sequences can grow or shrink very quickly because of this multiplying factor.
Besides arithmetic and geometric sequences, there are other types with different rules. Some might add a changing number each time or combine two previous terms to get the next one. These can be a bit trickier but are also more interesting since they don’t follow a single predictable pattern. For instance, the sequence 1, 1, 2, 3, 5, 8 adds the two previous terms together to get the next term.
USING THE NTH TERM
Understanding sequences is just the start. Knowing how to use formulas to find specific terms makes you a problem-solving pro.

FINDING THE NTH TERM
For arithmetic sequences, the formula to find the nth term is a + (n – 1)d. Here, ‘a‘ stands for the first term, and ‘d‘ is the common difference between terms. Suppose the first term of a sequence is 5 and the common difference is 3. Using the formula, the 10th term would be 5 + (10 – 1) × 3, which equals 32.
Geometric sequences use a different formula: ar^(n – 1). In this case, ‘a‘ is the first term and ‘r‘ is the common ratio. For instance, if the first term is 2 and the common ratio is 3, the 5th term would be 2 × 3^(5 – 1), giving us 162. This formula is especially helpful when dealing with larger numbers, as it quickly calculates the desired term.
Quadratic sequences add another layer of complexity. Their nth term is found using the formula an^2 + bn + c, where a, b, and c are constants. If a quadratic sequence starts with the terms 1, 4, 9, and you know the constants, it’s straightforward to plug them into the formula to find whichever term you need.

CONCLUSION
Putting these formulas to use can seem tricky at first, but with practice, it becomes second nature. Working out terms using their position in the sequence helps in many mathematical scenarios, from exam questions to real-life applications like finances or tracking patterns in data. So grab your calculator, and start practicing these formulas to ace your GCSE sequences.


No responses yet